Binary Search Tree

What is Binary Search Tree ?

It is a node-based binary tree data structure which has the following properties:

The left subtree of a node contains only nodes with keys lesser than the node’s key.
The right subtree of a node contains only nodes with keys greater than the node’s key.
The left and right subtree each must also be a binary search tree.
There must be no duplicate nodes.

Inserting a Key

Searching a Key

To search a given key in Binary Search Tree, we first compare it with root, if the key is present at root, we return root.
If key is greater than root’s key, we recur for right subtree of root node.
Otherwise we recur for left subtree.

Implementation:

class Node:
    def __init__(self, val):
        self.val = val
        self.left = None
        self.right = None


class BST:
    def __init__(self):
        self.root = None

    def insert(self, key):
        self.root = self.insert_util(self.root, key)

    def insert_util(self, node, key):
        if node is None:
            return Node(key)
        elif key <= node.val:
            node.left = self.insert_util(node.left, key)
        else:
            node.right = self.insert_util(node.right, key)

        return node

    def print_inorder(self):
        self.print_inorder_util(self.root)
        print()

    def print_inorder_util(self, node):
        if node:
            self.print_inorder_util(node.left)
            print(node.val, end=" ")
            self.print_inorder_util(node.right)

    def search_key(self, key):
        return self.search_key_util(self.root, key)

    def search_key_util(self, node, key):
        if not node:
            return False
        elif node.val == key:
            return True
        elif key <= node.val:
            return self.search_key_util(node.left, key)
        else:
            return self.search_key_util(node.right, key)


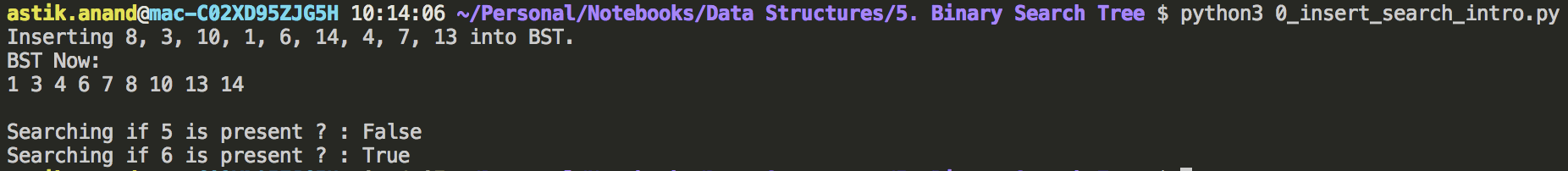
print("Inserting 8, 3, 10, 1, 6, 14, 4, 7, 13 into BST")
nodes = [8, 3, 10, 1, 6, 14, 4, 7, 13]
b = BST()
for key in nodes:
    b.insert(key)

print("BST Now:")
b.print_inorder()

print("Searching if 5 is present ? : {}".format(b.search_key(5)))
print("Searching if 6 is present ? : {}".format(b.search_key(6)))

Output:

bst_insert_search_output

Complexity:

Time: O(Logn) for both insert and search. In skewed tree it maybe O(n)
Auxilliary Space: O(1) if recursive call stack is not considered.

Some Interesting Facts:

Inorder traversal of BST always produces sorted output.
We can construct a BST with only Preorder or Postorder or Level Order traversal. Note that we can always get inorder traversal by sorting the only given traversal.
Number of unique BSTs with n distinct keys is Catalan Number

Applications of Binary Search Tree

Used to express arithmetic expressions.
Used to evaluate expression trees.
Used for managing virtual memory Areas (VMA’s).
Used for indexing IP addresses.
Hashing would be faster, but want to avoid attacker sending IP packets with worst-case inputs.
For dynamic sorting.

Standard BST Problems

1. Delete Node in BST***

When we delete a node, three possibilities arise.

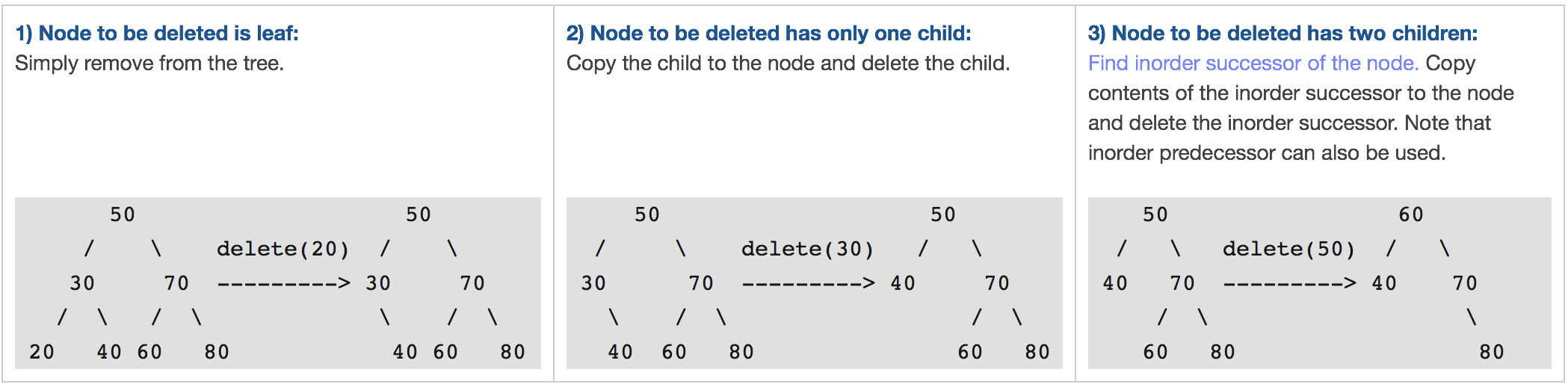
delete_node_in_bst

Algorithm:

Check if root None: key doesn’t exist, not possible to delete.
If key is lesser than root.val: Delete the key in left subtree.
If key is greater than root.val: Delete the key in right subtree.
If key is equal to root.val: Need to delete this root node.
- If no child exists: make root None and return None.
- If left child exists: make root None and return left child.
- If right child exists: make root None and return the right child.
- If both child exists:
  - Get the min_node from right child subtree.
  - Set the val of root as the val of min node.
  - Delete the min node from right subtree.
Finally return the root.

Implementation:

class Node:
    def __init__(self, val):
        self.val = val
        self.left = None
        self.right = None


def delete(root, key):
    # Check if root None: key doesn't exist, not possible to delete.
    if root is None:
        return root
    
    # If key is lesser than root.val: Delete the key in left subtree.
    if(key < root.val):
        root.left = delete(root.left, key)
    # If key is greater than root.val: Delete the key in right subtree.
    elif(key > root.val):
        root.right = delete(root.right, key)
    # If key is equal to root.val: Need to delete this root node.
    else:
        # If no child exists: make root None and return None.
        if(root.left is None and root.right is None):
            root = None
            return None
        # If left child exists: make root None and return left child.
        elif(root.right is None):
            temp = root.left
            root = None
            return temp
        # If right child exists: make root None and return the right child.
        elif(root.left is None):
            temp = root.right
            root = None
            return temp
        # If both child exists:
        else:
            # Get the min_node from right child subtree.
            temp = min_node(root.right)
            # Set the val of root as the val of min node.
            root.val = temp.val
            # Delete the min node from right subtree.
            root.right = delete(root.right, temp.val)
    
    # Finally return the root.
    return root


def min_node(current_node):
    # If current_node is None, min_node not possible
    if(current_node is None):
        return None
    
    min_node = current_node
    while(min_node.left):
        min_node = min_node.left
    
    return min_node


def insert(root, key):
    if(root is None):
        root = Node(key)
    
    # If key is lesser than root.val insert key in left subtree
    if(key <= root.val):
        if(root.left is None):
            root.left = Node(key)
        else:
            insert(root.left, key)
    # If key is greater than root.val insert key in right subtree
    else:
        if(root.right is None):
            root.right = Node(key)
        else:
            insert(root.right, key)


def print_bst_inorder(root):
    if(root):
        print_bst_inorder(root.left)
        print(root.val, end=" ")
        print_bst_inorder(root.right)
    


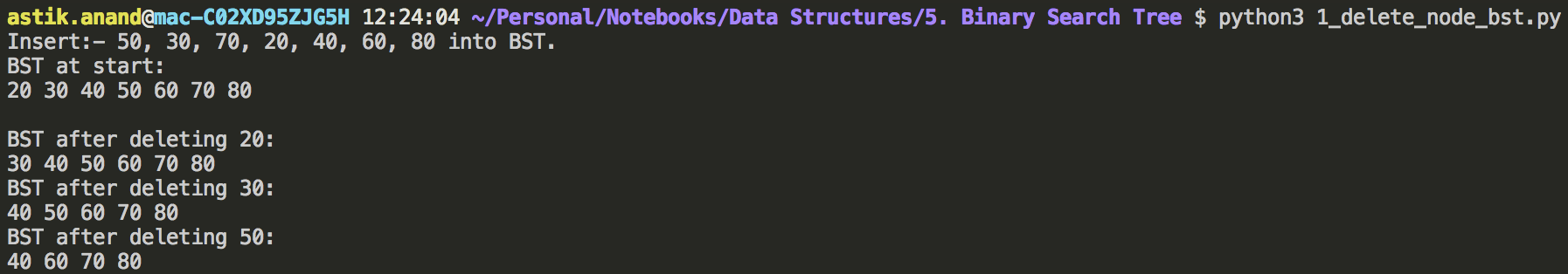
print("Insert:- 50, 30, 70, 20, 40, 60, 80 into BST.")
root = Node(50)
insert(root, 30)
insert(root, 70)
insert(root, 20)
insert(root, 40)
insert(root, 60)
insert(root, 80)
print("BST at start:")
print_bst_inorder(root)
print("\n")

delete(root, 20)
print("BST after deleting 20:")
print_bst_inorder(root)
print()

delete(root, 30)
print("BST after deleting 30:")
print_bst_inorder(root)
print()

delete(root, 50)
print("BST after deleting 50:")
print_bst_inorder(root)
print()

Output:

delete_node_in_bst_output

Complexity:

Time: O(Logn) for both insert and search. In skewed tree it maybe O(n)
Auxilliary Space: O(1) if recursive call stack is not considered.

2. Check if Binary Tree is BST***

Problem:

Given a binary tree check if it is a binary search tree.

Approach-1: Simple but Wrong X

For each node, check if left node is smaller and right node is greater.

Approach-2: Correct but Inefficient

For each node, check if max value in left subtree is smaller than the node and min value in right subtree greater than the node.
It runs slowly since it traverses over some parts of the tree many times and hence is inefficient.

Approach-3: Correct and Efficient

Approach-2 runs slowly since it traverses over some parts of the tree many times.
A better solution looks at each node only once.
The trick is to write a utility helper function isBSTUtil(struct node* node, int min, int max) that traverses down the tree keeping track of the narrowing min and max allowed values as it goes, looking at each node only once.
The initial values for min and max should be INT_MIN and INT_MAX — they narrow from there.

Approach-4: Inorder Traversal Method

Do In-Order Traversal of the given tree and store the result in a temp array.
Check if the temp array is sorted in ascending order, if it is, then the tree is BST.
We can also avoid the use of Auxiliary Array.
- While doing In-Order traversal, we can keep track of previously visited node.
- If the value of the currently visited node is less than the previous value, then tree is not BST.

Implementation (Approach-3):

import sys
INT_MIN = -sys.maxsize-1
INT_MAX = sys.maxsize

class Node:
    def __init__(self, val):
        self.val = val
        self.left = None
        self.right = None


def is_bst(root): 
    return (is_bst_util(root, INT_MIN, INT_MAX)) 
  

def is_bst_util(root, min_val, max_val): 
    # An empty tree is BST 
    if root is None: 
        return True
  
    # If current root's val is either less than min_val allowed or greater than max_val allowed return False.
    if root.val < min_val or root.val > max_val: 
        return False
  
    # Check the subtrees recursively tightening the min or max constraint 
    return (is_bst_util(root.left, min_val, root.val-1) and is_bst_util(root.right, root.val+1, max_val)) 


def print_tree(root):
    if(root):
        print_tree(root.left)
        print(root.val, end=" ")
        print_tree(root.right)
   


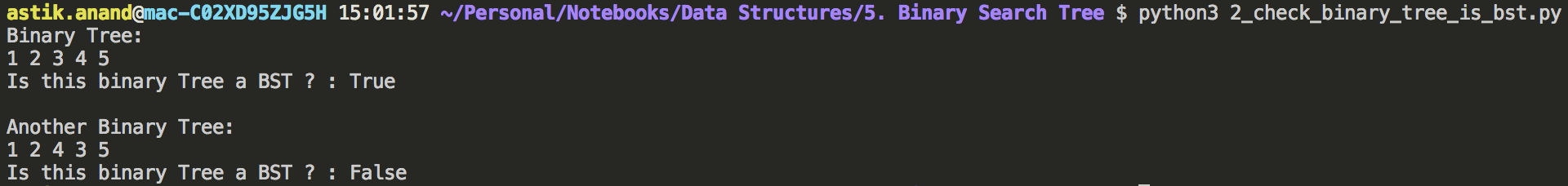
root = Node(4) 
root.left = Node(2) 
root.right = Node(5) 
root.left.left = Node(1) 
root.left.right = Node(3)
print("Binary Tree:")
print_tree(root)
print()
print("Is this binary Tree a BST ? : {}".format(is_bst(root)))

root = Node(3)
root.left = Node(2) 
root.right = Node(5) 
root.left.left = Node(1) 
root.left.right = Node(4)
print("\nAnother Binary Tree:")
print_tree(root)
print()
print("Is this binary Tree a BST ? : {}".format(is_bst(root)))

Output:

check_binary_tree_bst_output

Complexity:

Time: O(n)
Auxilliary Space: O(1) if recursive call stack is not considered.

3. Lowest Common Ancestor in BST (LCA)***

Problem:

Given values of two values n1 and n2 in a Binary Search Tree, find the Lowest Common Ancestor (LCA).

We may assume that both the values exist in the tree.

Approach:

If both key1 and key2 is smaller than root’s val, then lca exist in left subtree.
If both key1 and key2 is greater than root’s val, then lca exist in right subtree.
Else this root is LCA.

Implementation:

class Node:
    def __init__(self, val):
        self.val = val
        self.left = None
        self.right = None


def lowest_common_ancestor(root, key1, key2):
    while(root):
        # If both key1 and key2 is smaller than root's val, then lca exist in left subtree.
        if(key1 < root.val and key2 < root.val):
            root = root.left
        # If both key1 and key2 is greater than root's val, then lca exist in right subtree.
        elif(key1 > root.val and key2 > root.val):
            root = root.right
        # Else this root is LCA.
        else:
            break
    
    return root.val
        


root = Node(20) 
root.left = Node(8) 
root.right = Node(22) 
root.left.left = Node(4) 
root.left.right = Node(12) 
root.left.right.left = Node(10)
root.left.right.right = Node(14) 
print("Lowest Common Ancestor of 10 and 14 is : {}".format(lowest_common_ancestor(root, 10, 14)))
print("Lowest Common Ancestor of 14 and 8 is : {}".format(lowest_common_ancestor(root, 14, 8)))
print("Lowest Common Ancestor of 10 and 22 is : {}".format(lowest_common_ancestor(root, 10, 22)))

Output:

lca_in_bst_output

Complexity:

Time: O(n)
Auxilliary Space: O(1)

4. Inorder Successor in BST***

Problem:

Inorder successor of a node is the next node in Inorder traversal of the Binary Tree.
Inorder Successor is NULL for the last node in Inoorder traversal.
Inorder Successor of an input node can also be defined as the node with the smallest key greater than the key of input node.
So, it is sometimes important to find next node in sorted order.

Approach:

The Algorithm is divided into two cases on the basis of right subtree of the input node being empty or not.

If right subtree of given_node is NOT NULL: then successor lies in right subtree.
- Go to right subtree and return the node with minimum key value in right subtree.
If right subtree of given_node is NULL: then start from root and use search like technique.
- Travel down the tree, if a node’s data > root’s data then go right side, otherwise go to left side.

Algorithm:

Get the given node by search using key.
If given_node’s right exist, simply return the min_node from right.
Else: Set successor as None and start from root and search for successor by travelling down the tree.

given_node हमेशा successor के left subtree में होना चाहिए, इसलिए successor तभी update करेंगे जब left subtree में जाएंगे ।
- If given_node’s data < root’s data then go left side and update the successor.
- Else if a given_node’s data < root’s data then go to right side.
- Else break when given_node’s data and root’s data are equal, given_node is found.
Finally return the successor.

Implementation:

class Node:
    def __init__(self, val):
        self.val = val
        self.left = None
        self.right = None


def inorder_successor(root, key):
    # Get the given node by search using key.
    given_node = search(root, key)

    # If given_node's right exist, simply return the min_node from right.
    if(given_node.right):
        return min_node(given_node.right)
    
    # Set successor as None and start from root and search for successor by travelling down the tree.
    successor = None
    while(root):
        # If given_node’s data < root’s data then go left side and update the successor.
        # given_node हमेशा successor के left subtree में होना चाहिए, 
        # इसलिए successor तभी update करेंगे जब left subtree में जाएंगे ।
        if(given_node.val < root.val):
            successor = root
            root = root.left
        # Else if a given_node’s data > root’s data then go to right side.
        elif (given_node.val > root.val):
            root = root.right
        # Else break when given_node’s data and root’s data are equal, given_node is found.
        else:
            break
    
    # Finally return the successor.
    return successor

        
def search(root, key):
    # If root is None, then key doesn't exist.
    if root is None:
        return root
    
    # If root's val matches the key, then we have found the key in 
    if(root.val == key):
        return root
    
    # If key is lesser than root's val search in left subtree else serach in right subtree.
    if(key < root.val):
        return search(root.left, key)
    else:
        return search(root.right, key)


def min_node(current_node):
    # If current_node is None, min_node not possible
    if(current_node is None):
        return None
    
    min_node = current_node
    while(min_node.left):
        min_node = min_node.left
    
    return min_node



root = Node(20) 
root.left = Node(8) 
root.right = Node(22) 
root.left.left = Node(4) 
root.left.right = Node(12) 
root.left.right.left = Node(10)
root.left.right.right = Node(14) 
print("Inorder Successor of  8 is : {}".format(inorder_successor(root, 8).val))
print("Inorder Successor of 10 is : {}".format(inorder_successor(root, 10).val))
print("Inorder Successor of 14 is : {}".format(inorder_successor(root, 14).val))

Output:

inorder_successor_bst_output

Complexity:

Time: O(Logn)
Auxilliary Space: O(1)

← Previous: Binary Tree Next: Heap →

Binary Search Tree

What is Binary Search Tree ?

Inserting a Key

Searching a Key

Implementation:

Complexity:

Some Interesting Facts:

Applications of Binary Search Tree

Standard BST Problems

1. Delete Node in BST***

Algorithm:

Implementation:

Complexity:

2. Check if Binary Tree is BST***

Problem:

Approach-1: Simple but Wrong X

Approach-2: Correct but Inefficient

Approach-3: Correct and Efficient

Approach-4: Inorder Traversal Method

Implementation (Approach-3):

Complexity:

3. Lowest Common Ancestor in BST (LCA)***

Problem:

Approach:

Implementation:

Complexity:

4. Inorder Successor in BST***

Problem:

Approach:

Algorithm:

Implementation:

Complexity:

Subscribe !

Connect to me through Social Networks !

Contact Me !