Pattern Search Algorithms

Why Pattern Search Algorithms ?

Many a times we need to find a pattern pat[0..m-1] in a given text txt[0..n-1] assuming n >= m.
In those situation we use pattern search algorithms.
Example:

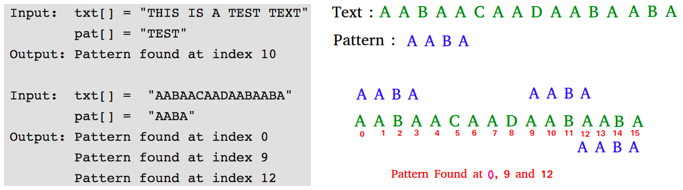
pattern_search_introduction

Pattern searching is an important problem in computer science.
When we do search for a string in notepad/word file or browser or database, pattern searching algorithms are used to show the search results.

Famous Pattern Search Algorithms

Naive Algorithm
Rabin-Karp Algorithm
KMP (Knuth-Morris-Pratt) Algorithm

Algo-1: Naive Algorithm

Just need to use 2 loops to check every substring of same length as of given pattern and check if it matches it.

Implementation:

# Given a text txt[0..n-1] and a pattern pat[0..m-1], write a function search(pat, txt) that prints all occurrences of pat[] in txt[]. 
# You may assume that n > m.


def naive_pattern_search(pat, text):
    m = len(pat)
    n = len(text)

    for i in range(n-m+1):
        flag = True
        for j in range(m):
            if(pat[j] != text[i+j]):
                flag = False
        
        if(flag):
            print("Pattern found at index {}".format(i))


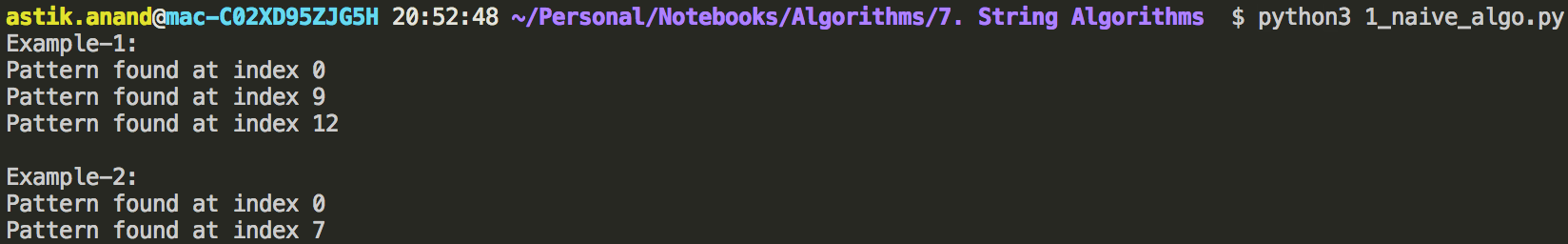
print("Example-1:")
txt = "AABAACAADAABAABA"
pat = "AABA"
naive_pattern_search(pat, txt)

print("Example-2:")
txt = "abracadabra"
pat = "ab"
naive_pattern_search(pat, txt)


# Time Complexity:
# Best Case: O(n) - First character of pattern is not present in text
# Worst Case: O(n-m+1)(m) - All characters of the text and pattern are same OR only the last character is different.

Output:

pattern_search_naive_algo_output

Complexity:

Time:
- Best Case: O(n) - First character of pattern is not present in text
- Worst Case: O(n-m+1)(m) - All characters of the text and pattern are same OR only the last character is different.
Auxilliary Space: O(1)

Algo-2: Rabin-Karp Algorithm***

Like the Naive Algorithm, Rabin-Karp algorithm also slides the pattern one by one.
But unlike the Naive algorithm, Rabin Karp algorithm matches the hash value of the pattern with the hash value of current substring of text,and if the hash values match then only it starts matching individual characters.
Rabin-Karp algorithm needs to calculate hash values for following strings:
1. Pattern itself.
2. All the substrings of text of length m.
Calculating hash function as suggested by Rabin-Karp:
- We treat string character with its ASCII value and hence we take base as 256.
- The numeric values cannot be practically stored as an integer.
- Hence numeric value is calculated using modular arithmetic to make sure that the hash values can be stored in an integer variable.
- To do rehashing, we need to take off the most significant digit and add the new least significant digit for hash value.
Rehashing Formula:

hash( txt[s+1 .. s+m] ) = ( d ( hash(txt[s .. s+m-1]) – txt[s]*h ) + txt[s + m] ) mod q

Implementation:

def rabin_karp_search(pat, text):
    base = 256         # Total number of characters, if you consider less numbers of characters 
                       # base can be set accordingly
    q = 101            # Prime to take modulo
    p_hash = 0         # Hash value for pattern
    t_hash = 0         # Hash value for text
    m = len(pat)
    n = len(text)

    # Calculating h that will be used for rehashing:  h = pow(base, m-1)%q
    h = 1
    for i in range(m-1):
        h = (h*base)%q
    
    # Calculating hash for pat and initial text
    for i in range(m):
        p_hash = (p_hash*base + ord(pat[i]))%q      # ord(char) : gives ascii value of char
        t_hash = (t_hash*base + ord(text[i]))%q

    # Slide the pattern over text one by one 
    for i in range(n-m+1):
        # Check the hash values of current window of text and pattern
        if t_hash == p_hash:   # if the hash values match then only check for characters one by one 
            for j in range(m):
                if(text[i+j] != pat[j]):
                    break
            if(j==m-1):
                print("Found at index {}".format(i))
        
        # Calculate hash value for next window of text: Remove leading digit, add trailing digit 
        if i+m < n:
            t_hash = ((t_hash - h*ord(text[i]))*base + ord(text[i+m]))%q

            # We might get negative values of t_hash, converting it to positive 
            if t_hash < 0: 
                t_hash = t_hash+q 


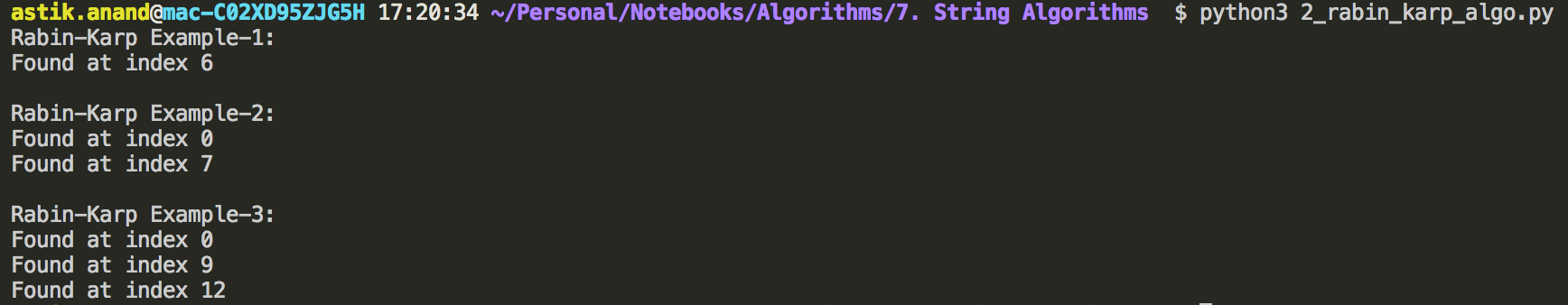
print("Rabin-Karp Example-1:")
txt = "bacbabababacaca"
pat = "ababaca"
rabin_karp_search(pat, txt)

print("\nRabin-Karp Example-2:")
txt = "abracadabra"
pat = "ab"
rabin_karp_search(pat, txt)

print("\nRabin-Karp Example-3:")
txt = "AABAACAADAABAABA"
pat = "AABA"
rabin_karp_search(pat, txt)

# Complexity:
# Time: O(n)
# Auxilliary Space: O(1)

Output:

rabin_karp_output

Complexity:

Time:
- Average & Best Case: O(n + m)
- Worst Case: O(n)(m) - Failure of hash function that leads to all spurious hits
Auxilliary Space: O(1)

Algo-3: KMP (Knuth Morris Pratt) Algorithm***

Naive doesn’t work well in cases where we see many matching characters followed by a mismatching character.
Example: txt = “AAAAAAAAAAAAAAAAAB” and pat = “AAAAB”
KMP algorithm uses degenerating property (pattern having same sub-patterns appearing more than once in the pattern) of the pattern & improves the worst case complexity to O(n).
The basic idea behind KMP’s algorithm is: whenever we detect a mismatch (after some matches), we already know some of the characters in the text of the next window.
We take advantage of this information to avoid matching the characters that we know will anyway match.

Step-1: Preprocessing

KMP algorithm preprocesses pat[] and constructs an auxiliary lps[] of size m (same as size of pattern) which is used to skip characters while matching.
Name lps indicates longest proper prefix which is also suffix.
A proper prefix is prefix with whole string notallowed.

Example:

Prefixes of “ABC” are “ “, “A”, “AB” and “ABC”.

Proper prefixes are “ “, “A” and “AB”.

Suffixes of the string are “ “, “C”, “BC” and “ABC”.
We search for lps in sub-patterns. More clearly we focus on sub-strings of patterns that are either prefix and suffix.
For each sub-pattern pat[0..i] where i = 0 to m-1, lps[i] stores length of the maximum matching proper prefix which is also a suffix of the sub-pattern pat[0..i].
For each sub-pattern pat[0..i] where i = 0 to m-1, lps[i] stores length of the maximum matching proper prefix which is also a suffix of the sub-pattern pat[0..i].

lps[i] = the longest proper prefix of pat[0..i] which is also a suffix of pat[0..i]

Longest Proper Prefix also a Suffix: LPS[]

Calculating LPS or Pi (π) or False function

Algorithm to calculate π

Start from j=0, i=1 and π[0]=0 for pat.
if pat[i]==pat[j] put π[i]=j+1 and increase both i and j.
Else if it doesn’t match and j>0 change j = π[j-1].
Else if j==0 put π[i]=0 and increase i.

Implementation to calculate π

def calculate_lps(pat):
    m = len(pat)
    lps = [0]*m
    i = 1; j = 0

    while(i < m):
        if(pat[i] == pat[j]):
            lps[i] = j+1
            i+=1
            j+=1
        elif(j>0):
            j = lps[j-1]
        else:
            lps[i] = 0
            i+=1
    
    return lps


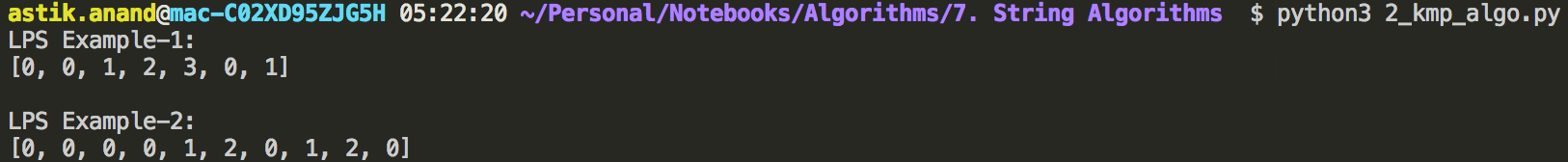
print("LPS Example-1:")
pat = "ababaca"
print(str(calculate_lps(pat)))

print("\nLPS Example-2:")
pat = "abcdabeabf"
print(str(calculate_lps(pat)))

# Complexity:
# Time: O(m)
# Auxilliary Space: O(m)

Output:

calculate_lps

Complexity:

Time: O(m)
Auxilliary Space: O(m)

Step-2: Matching Algorithm

Start from i=0, j=0 we will use i to track text and j to track pat.
if text[i] == pat[j] then increase both i and j and check
- if j==m then it’s match print and reset j = lps[j-1] to find next occurrence of the pattern
Else if it doesn’t match and j>0 change j = lps[j-1].
Else if j==0 then increase i.

Implementation

def KMP_search(pat, text):
    lps = calculate_lps(pat)
    i=0; j=0
    m = len(pat)
    n = len(text)

    while(i<n):
        if(text[i] == pat[j]):
            i+=1
            j+=1
            if(j == m):
                print("Found at index {}".format(i-j))
                j = lps[j-1]
        elif(j>0):
            j = lps[j-1]
        else:
            i+=1

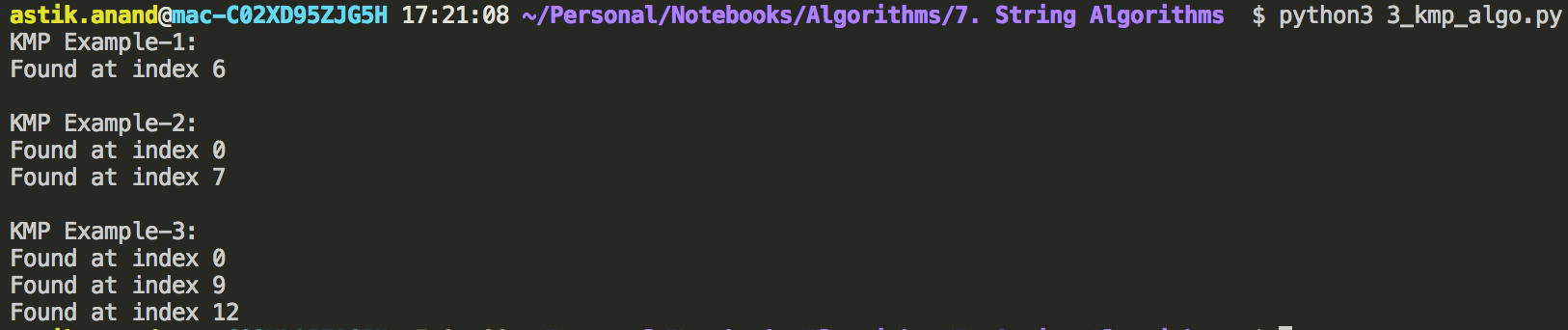
print("KMP Example-1:")
txt = "bacbabababacaca"
pat = "ababaca"
KMP_search(pat, txt)

print("\nKMP Example-2:")
txt = "abracadabra"
pat = "ab"
KMP_search(pat, txt)

print("\nKMP Example-3:")
txt = "AABAACAADAABAABA"
pat = "AABA"
KMP_search(pat, txt)

# Complexity:
# Time: O(m+n)
# Auxilliary Space: O(m)

Output:

kmp_algo_output

Complexity:

Time: O(n+m)
Auxilliary Space: O(m)

Standard Pattern Search Algorithms Problmes

1. Anagram Substring Search

Problem:

Given a text txt[0..n-1] and a pattern pat[0..m-1].

Write a function search(pat, txt) that prints all occurrences of pat[] and its permutations (or anagrams) in txt[].

You may assume that n > m.

Algorithm:

Store counts of frequencies of pattern in first count array countP[] and counts of frequencies of characters in first window of text in array countTW[].
Now run a loop from i =m to n-1. Do following in loop.
- a) If the two count arrays are identical, we found an occurrence.
- b) Increment count of current character of text in countTW[]
- c) Decrement count of first character in previous window in countWT[]
The last window is not checked by above loop, so explicitly check it.

Implementation

def anagram_search(pat, text):
    m = len(pat)
    n = len(text)
    count_pat = [0]*256     # Total 256 characters
    count_text_window = [0]*256

    # fill the count_pat and count_text_window array
    for i in range(m):
        count_pat[ord(pat[i])] += 1
        count_text_window[ord(pat[i])] += 1
    
    # Starts searching anagrams or permutations
    for i in range(m, n):
        if(count_pat == count_text_window):
            print("Found at index {}".format(i-m))
        
        # Increase the frequency of next character and decrease the frequncy of first character in window
        count_text_window[ord(text[i])] += 1
        count_text_window[ord(text[i-m])] -= 1
    
    # Check for last window as it will be left in this loop
    if(count_pat == count_text_window):
            print("Found at index {}".format(n-m))


print("Anagram Search Example-1:")
txt = "BACDGABCDA"
pat = "ABCD"       
anagram_search(pat, txt)

Output:

anagram_search_output

Complexity:

Time: O(n)
Auxilliary Space: O(1) : Only 256 spaces can be treated as constant.

2. Manacher’s Algorithm - O(N) Time Longest Palindromic Substring***

Problem:

Given a string, find the longest substring which is palindrome.

Examples:

string = “abaabc” output = “baab”

string = “babcbabcbaccba” output = “abcbabcba”

string = “abaaba” output = “abaaba”

string = “abababa” output = “abababa”

string = “forgeeksskeegfor” output = “geeksskeeg”

Approaches to solve:

Naive Approach : O(n³)
Dynamic Programming: O(n²)
Manacher Algorithm: O(n)

Manacher Approach:

manacher_approach

Manacher Algorithm:

Pre-Processing:
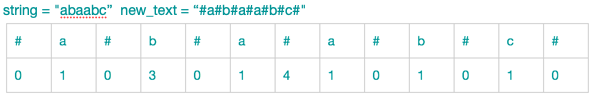
- Push ’#’ before and after every character and create a new_text. Example: text = “abc” then new_text= “#a#b#a#”
- Create an array P[] of size(2n+1) to store palindromic values of the new_text.
- Initialize Center(C) = 0 and Right(R) = 0
Processing:
- Loop over the pre-processed new_text and do
- Get mirror_index of currently processing value(i) using center(C).
- Check if Right(R) is greater than current(i): then put min(R-i, P[i_mirror]) or else put 0 in P[i].
- Keep expanding using current and already palindrome (at that stage using p[I]) until the next character and mirror character from i are same.
- Now if newly calculated P[i] is greater than R-i then update C=i and R=P[i] + i
Post-Processing:
- Find the max of P[] array and that is the max_length of palindrome and its index is center_index
- Calculate start and end index of palindrome in original text using start = (center_index-max_pal_len)/2 and end = (center_index+max_pal_len)/2
- Print the palindrome using start and end index using original text.

Implementation

def manacher_longest_palindromic_substring(text):
    # Preprocess the given text
    new_text = "#"
    for ch in text:
        new_text += ch
        new_text += "#"
    
    n = len(new_text)
    P = [0]*n    # Array to store palindromic values

    # Center(C) : Index of mirror line
    # Right(R)  : Where we are going to end our bounds on right hand side
    # Left(L)   : No need to store Left(L) as it can be calculated using something like i_mirror
    C = 0
    R = 0

    # Iterate through entire new_text
    for i in range(n):
        # i is pointing to current element
        mirror = C - (i-C)  # Mirror index of i

        # If i < right bound(R) then simply copy min of mirror value or (R-i)
        if(i < R):
            P[i] = min(R-i, P[mirror])

        # Expand around both sides of i to find new center(C)
        while(((i + P[i] + 1) < n) and ((i - P[i] - 1) >= 0) and \
              (new_s[i + P[i] + 1] == new_s[i - P[i] - 1])):
            P[i] += 1

        # If i + P[i] > right bound(R) then update Center and Right bound
        if(i + P[i] > R):
            C = i
            R = i + P[i]

    # Find the length of largest palindrome and center_index
    max_pal_len = max(P)
    center_index = P.index(max_pal_len)

    # Print the longest palindrome
    start = int((center_index-max_pal_len)/2)
    end = int((center_index+max_pal_len)/2)

    print("Largest Palindromic Susbtring: {}".format(text[start:end]))




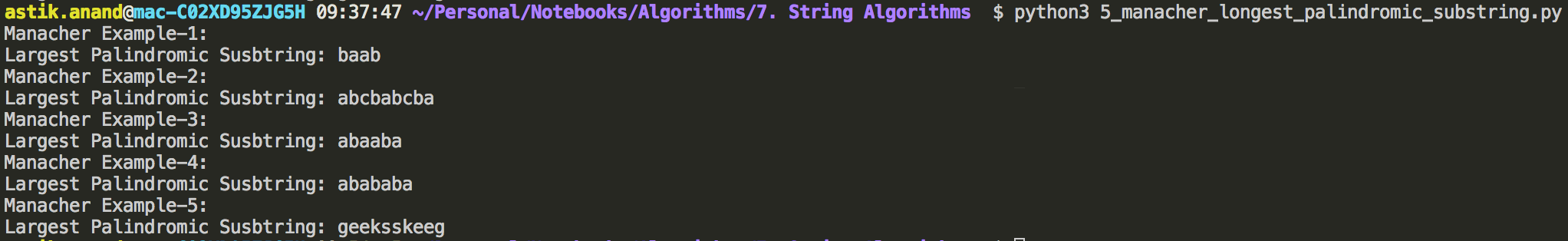
print("Manacher Example-1:")
txt = "abaabc"
manacher_longest_palindromic_substring(txt)

print("Manacher Example-2:")
txt = "babcbabcbaccba"
manacher_longest_palindromic_substring(txt)

print("Manacher Example-3:")
txt = "abaaba"
manacher_longest_palindromic_substring(txt)

print("Manacher Example-4:")
txt = "abababa"
manacher_longest_palindromic_substring(txt)

print("Manacher Example-5:")
txt = "forgeeksskeegfor"
manacher_longest_palindromic_substring(txt)

Output:

manacher_algo_output

Complexity:

Time: O(n) : Traversing through loop only once.
Auxilliary Space: O(n) : We are using (2n+1) spaces array

3. All Possible Strings - Made by Placing Spaces***

Problem:

Given a string, print all possible strings that can be made by placing spaces (zero or one) in between them.

Example:

input = ABC

output:

ABC

AB C

A BC

A B C

Algorithm:

Use recursion and create a buffer that one by one contains all output strings having spaces.
Keep updating buffer in every recursive call.
If the length of given string is “n” the updated string can have maximum length of 2n-1 and so we create buffer size of 2n (one extra character for string termination).
Leave 1st character as it is, starting from the 2nd character, either fill a space or a character.

Implementation:

def to_string(str_list): 
    s = "" 
    for x in str_list: 
        if x == "$": 
            break
        s += x 
    return s 


def print_pattern_util(string, buffer, i, j, n):
    if(i==n):
        buffer[j] = "$"
        print(to_string(buffer))
        return
    
    # Either put the character 
    buffer[j] = string[i] 
    print_pattern_util(string, buffer, i+1, j+1, n) 
  
    # Or put a space followed by next character 
    buffer[j] = " "
    buffer[j+1] = string[i] 
  
    print_pattern_util(string, buffer, i+1, j+2, n)
    


def print_pattern(string):
    n = len(string)
    buffer = [0]*(2*n)
    buffer[0] = string[0]

    print_pattern_util(string, buffer, 1, 1, n)



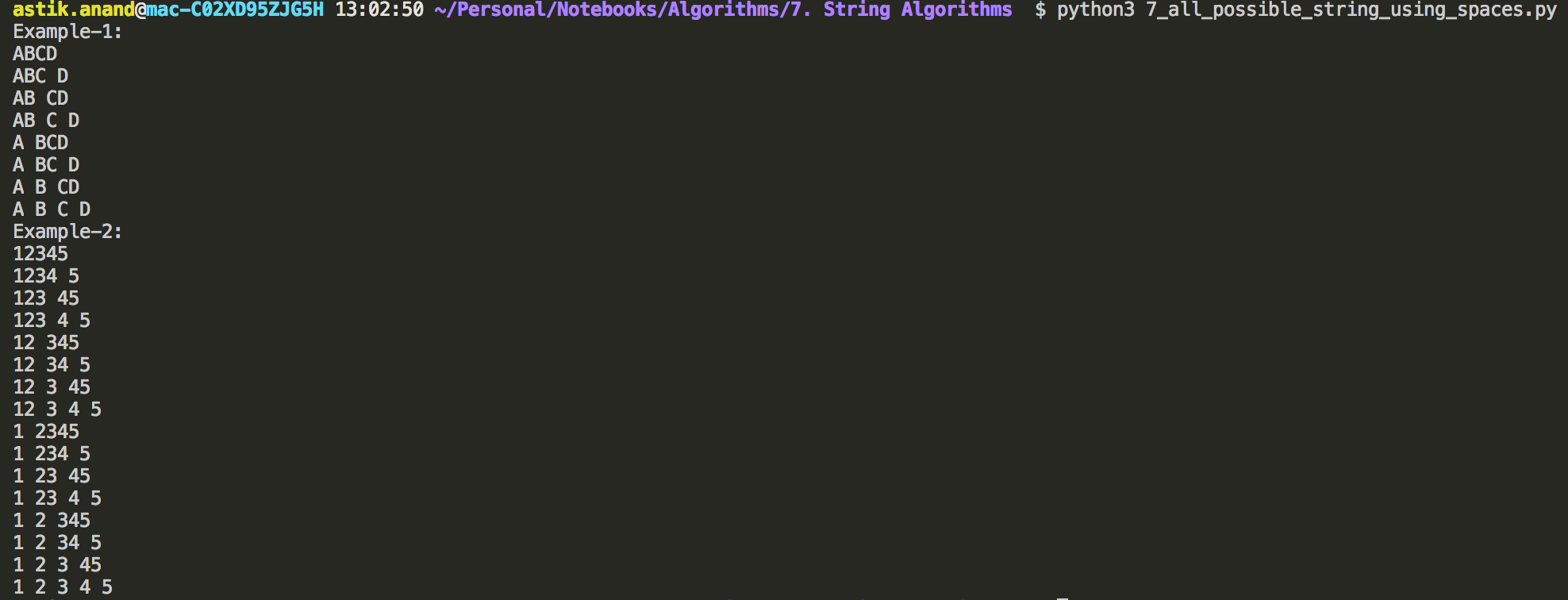
print("Example-1:")
string = "ABCD"
print_pattern(string)

print("Example-2:")
string = "12345"
print_pattern(string)

Output:

all_possible_strings_output

Complexity:

Time: O(n*(2ⁿ)) : Since number of Gaps are n-1, there are total 2^n-1 patterns each having length ranging from n to 2n-1, hence overall complexity would be O(n*(2ⁿ))
Auxilliary Space: O(n) : We are using (2n) spaces array

Problems To Do:

Longest Even Length Substring :- Sum of 1st & 2nd half is same
Print all Anagrams Together - Sequence of words are given

← Previous: Divide and Conquer Approach

Next: Bit Algorithms →

Pattern Search Algorithms

Why Pattern Search Algorithms ?

Algo-1: Naive Algorithm

Implementation:

Complexity:

Algo-2: Rabin-Karp Algorithm***

Implementation:

Complexity:

Algo-3: KMP (Knuth Morris Pratt) Algorithm***

Step-1: Preprocessing

Implementation to calculate π

Complexity:

Step-2: Matching Algorithm

Implementation

Complexity:

Standard Pattern Search Algorithms Problmes

1. Anagram Substring Search

Problem:

Algorithm:

Implementation

Complexity:

2. Manacher’s Algorithm - O(N) Time Longest Palindromic Substring***

Problem:

Approaches to solve:

Manacher Approach:

Manacher Algorithm:

Implementation

Complexity:

3. All Possible Strings - Made by Placing Spaces***

Problem:

Algorithm:

Implementation:

Complexity:

Problems To Do:

Subscribe !

Connect to me through Social Networks !

Contact Me !