Mathematical Algorithms

Algorithms deals with Mathematical calculations and its aspects.

Standard Mathematical Algorithm Problems

1. Lucky Numbers***

What are lucky numbers?

Take the set of integers

1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 14, 15, 16, 17, 18, 19,……

First, delete every second number, we get following reduced set.

1, 3, 5, 7, 9, 11, 13, 15, 17, 19,…………

Now, delete every third number, we get

1, 3, 7, 9, 13, 15, 19,….….

Continue this process indefinitely……

Any number that does NOT get deleted due to above process is called “lucky”.

Therefore, set of lucky numbers is 1, 3, 7, 13,………

Problem:

Given a number check if it is lucky or not.

Algorithm:

Before every iteration, if we calculate position of the given no, then in a given iteration, we can determine if the no will be deleted.
Suppose calculated position for the given no. is p before some iteration, and each ith number is going to be removed in this iteration,
- if p < i then input number is lucky,
- if p is such that p%i == 0 (i is a divisor of p), then input number is not lucky.

Implementation:

def check_lucky(n, k):
    # n is current_position of number
    # k represents at this iteration kth numbers will be deleted, at start k = 2

    if(n%k == 0):
        return False
    
    if(k > n):
        return True
    
    # Calculate new_position after deleting kth number, increase k
    n = n - int(n/k)
    k += 1

    return check_lucky(n, k)



print("Example-1: check_lucky : 5")
print(check_lucky(5, 2))

print("Example-2: check_lucky : 19")
print(check_lucky(19, 2))

Output:

lucky_numbers

Complexity:

Time: O(loglogn)

2. Square Root - Babylonian Method***

Problem:

Find approximate square root of a number.

Algorithm:

Start with an arbitrary positive start value x (the closer to the root, the better).
Initialize y = 1.
Do following until desired approximation is achieved.
- a) Get the next approximation for root using average of x and y
- b) Set y = n/x

Implementation:

def square_root(n):
    # Here using n itself as initial approximation but this can definitely be improved
    x = n
    y = 1

    # a decides the accuracy level 
    a = 0.000001

    while(x - y > a): 
        x = (x + y)/2
        y = n / x 
      
    return x



print("Example-1: square_root : 4")
print(round(square_root(4), 5))

print("Example-2: square_root : 50")
print(round(square_root(50), 5))

Output:

square_root

Complexity:

**Time: **

3. Sieve of Eratosthenes

The sieve of Eratosthenes is one of the most efficient ways to find all primes smaller than n when n is smaller than 10 million or so.

Problem:

Given a number n, print all primes smaller than or equal to n. It is also given that n is a small number.

Example:

Input : n = 10

Output : 2 3 5 7

Input : n = 20

Output: 2 3 5 7 11 13 17 19

Algorithm:

Create a list of consecutive integers from 2 to n: (2, 3, 4, …, n).
Initially, let p=2, the first prime number.
Starting from p2, count up in increments of p and mark each of these numbers greater than or equal to p2 itself in the list. These numbers will be p(p+1), p(p+2), p(p+3), etc..
Find the first number greater than p in the list that is not marked.
- If there was no such number, stop.
- Otherwise, let p now equal this number (which is the next prime), and repeat from step 3.

Implementation:

def sieve_of_eratosthenes(n):
    primes = [True]*(n+1)
    p = 2

    # Initialize all to be True, a value in prime[i] will finally be False if i is Not a prime, else True.
    while(p*p <= n):
        # If prime[p] is still True, then it is a prime 
        if(primes[p] == True):
            # Update all multiples of p staring from p*p, then p*(p+1), p*(p+2) and so on
            for i in range(p*p, n+1, p):
                primes[i] = False
        
        p += 1
    
    # Print all primes
    for i in range(2, n+1):
        if(primes[i]):
            print(i, end=" ")
    print()



print("Example-1: sieve_of_eratosthenes : 30")
sieve_of_eratosthenes(30)

print("\nExample-2: sieve_of_eratosthenes : 101")
sieve_of_eratosthenes(101)

Output:

sieve_of_erathosthenes

Complexity:

**Time: **

4. Next Smallest Palindrome***

Problem:

Given a number, find the next smallest palindrome larger than this number.

Examples:

Input: 999 Output: 1001

Input: 1234 Output: 1331

Input: 1213 Output: 1221

Input: 1221 Output: 1331

Input: 23921 Output: 23932

Input: 23941 Output: 24042

Implementation:

import math


def reverse(string):
    return string[::-1]


def all_9s(n):
    for digit in n:
        if(digit != "9"):
            return False
    return True


def next_smallest_palindrome(n):
    n = str(n)
    k = len(n)
    # If all digits are 9s then put 1(k-1 zeroes)1
    if(all_9s(n)):
        return "1"+str("0"*(k-1))+"1"
    
    # Get the left half
    left_half = n[:(int(math.ceil(k/2)))]
    # If the number of digits are even
    if(k%2==0):
        # Check if created palindrome i.e. pal = left_half + reverse(left_half) 
        # is lesser or equal to given number
        # If it is lesser or equal increment left_half by 1.
        if(left_half + reverse(left_half) <= n):
            left_half = str(int(left_half)+1)
        
        pal = left_half + reverse(left_half)
    # If number of digits are odd
    else:
        if(left_half + reverse(left_half[:-1]) <= n):
            left_half = str(int(left_half)+1)
        
        pal = left_half + reverse(left_half[:-1])

    return pal


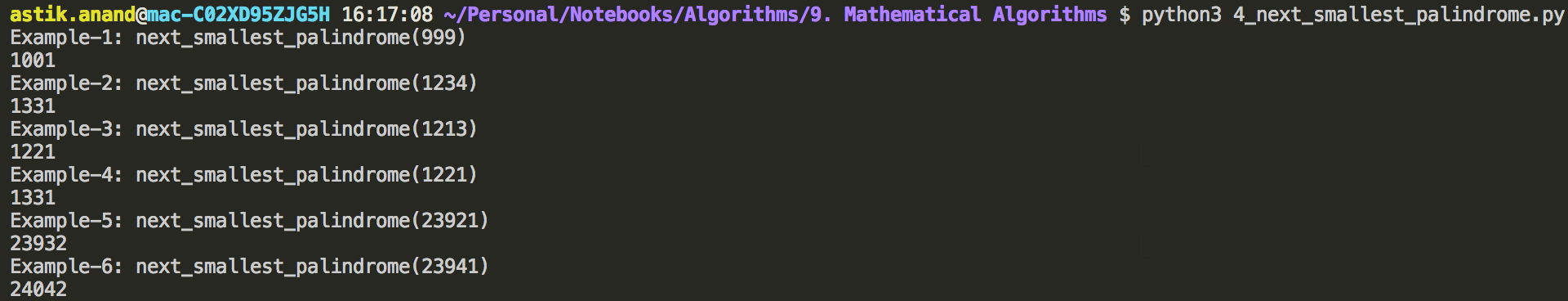
print("Example-1: next_smallest_palindrome(999)")
print(next_smallest_palindrome(999))

print("Example-2: next_smallest_palindrome(1234)")
print(next_smallest_palindrome(1234))

print("Example-3: next_smallest_palindrome(1213)")
print(next_smallest_palindrome(1213))

print("Example-4: next_smallest_palindrome(1221)")
print(next_smallest_palindrome(1221))

print("Example-5: next_smallest_palindrome(23921)")
print(next_smallest_palindrome(23921))

print("Example-6: next_smallest_palindrome(23941)")
print(next_smallest_palindrome(23941))

Output:

next_smallest_palindrome_output

Complexity:

**Time: O(n) **

5. Prime Factors

Problem:

Given a number n, write an efficient function to print all prime factors of n.

Examples:

Input: 12 Output: 2, 2, 3

Input: 315 Output: 3, 3, 5, 7

Algorithm:

While n is divisible by 2, print 2 and divide n by 2.
After step 1, n must be odd. Now start a loop from i = 3 to square root of n. While i divides n, print i and divide n by i, increment i by 2 and continue.
If n is a prime number and is greater than 2, then n will not become 1 by above two steps. So print n if it is greater than 2.

Implementation

import math

def prime_factors(n):
    # Check 2 as prime factors
    while(n%2 == 0):
        print(2, end=" ")
        n = int(n/2)
    
    # Check 3, 5, 7, 11, ... and so on as prime factors
    for i in range(3, int(math.sqrt(n))+1, 2):
        while(n%i==0):
            print(i, end=" ")
            n = int(n/i)
    

    # If n is a prime greater than 2
    if(n > 2):
        print(n)
    
    print()


print("Example-1: prime_factors(12)")
prime_factors(12)

print("Example-2: prime_factors(315)")
prime_factors(315)

Output:

prime_factors_output

Complexity:

**Time: O(n) **

6. Trailing Zeroes in Factorial

Problem:

Given an integer n, write a function that returns count of trailing zeroes in n!.

Examples:

Input: 5 Output: 1 coz 5! = 120 1 trailing zero

Input: 20 Output: 4 coz 20! = 2432902008176640000 4 trailing zeroes

Input: 100 Output: 24

Approach:

Trailing 0s in n! = Count of 5s in prime factors of n! = floor(n/5) + floor(n/25) + floor(n/125) + ….

Implementation:

def factorial_trailing_zeroes(n):
    count = 0
    k = 5
    while(n > 0):
        n = int(n/k)
        count += n
        k = k*k
    
    print(count)



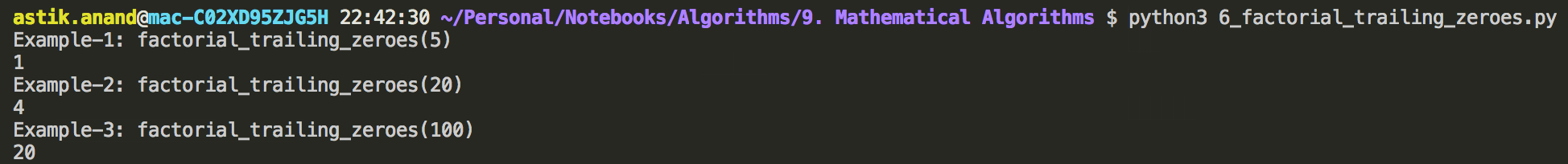
print("Example-1: factorial_trailing_zeroes(5)")
factorial_trailing_zeroes(5)

print("Example-2: factorial_trailing_zeroes(20)")
factorial_trailing_zeroes(20)

print("Example-3: factorial_trailing_zeroes(100)")
factorial_trailing_zeroes(100)

Output:

trailing_zeroes_factorial_output

Complexity:

**Time: O(loglogn) **

7. Next Greater with Same Digits***

Problem:

Given a number n, find the smallest number that has same set of digits as n and is greater than n.

If n is the greatest possible number with its set of digits, then print “not possible”.

Examples:

Input: 218765 Output: 251678

Input: 1234 Output: 1243

Input: 4321 Output: “Not Possible”

Input: 534976 Output: 536479

Algorithm:

Traverse the given number from rightmost digit, keep traversing till you find a digit which is smaller than the previously traversed digit.
- Example: if the input number is “534976”, we stop at 4 because 4 is smaller than next digit 9.
- If we do not find such a digit, then output is “Not Possible”.
Now search the right side of above found digit ‘d’ for the smallest digit greater than ‘d’.
- Example: In “534976″, the right side of 4 contains “976”. The smallest digit greater than 4 is 6.
Swap the above found two digits, we get 536974 in above example.
Now sort all digits from position next to ‘d’ to the end of number. The number that we get after sorting is the output.
- Example: In above, we sort digits in bold 536974. We get “536479” which is the next greater number for input 534976.

Implementation:

def next_greater_same_digits(n):
    n = list(str(n))
    k = len(n)

    # Finding a  digit which is smaller than the previously traversed digit
    for i in range(k-1, -1, -1):
        if(n[i-1] < n[i]):
            break
    
    if(i==0):
        return "Not possible"
    
    # Find the smallest digit on the right side of (i-1)'th digit that is greater than x 
    x = n[i-1]
    smallest = i
    for j in range(i+1, k):
        if(n[j] < n[smallest] and  n[j] > x):
            smallest = j
    
    # Swapping the above found smallest digit with (i-1)'th
    n[i-1], n[smallest] = n[smallest], n[i-1]

    # Sort all the digits from ith digit and concatenate it with left_part
    result_num = n[:i] + sorted(n[i:])

    # Join all the array element to create a number
    result_num = "".join(result_num)

    return result_num

    

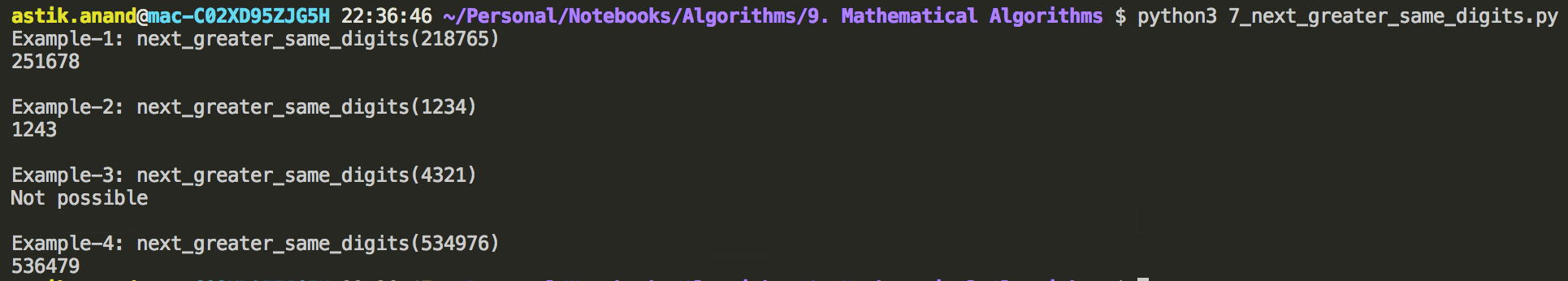
print("Example-1: next_greater_same_digits(218765)")
print(next_greater_same_digits(218765))

print("\nExample-2: next_greater_same_digits(1234)")
print(next_greater_same_digits(1234))

print("\nExample-3: next_greater_same_digits(4321)")
print(next_greater_same_digits(4321))

print("\nExample-4: next_greater_same_digits(534976)")
print(next_greater_same_digits(534976))

Output:

next_greater_same_digits

Complexity:

Time: O(size)

8. Clock Angle Problem***

Problem:

Find angle between hands of an analog clock at a given time.

Examples:

Input: h = 9:00, m = 60.00 Output: 90 degree

Input: h = 12:00, m = 30.00 Output: 165 degree

Input: h = 3.00, m = 30.00 Output: 75 degree

Approach:

The idea is to take 12:00 (h = 12, m = 0) as a reference. Then

Calculate the angle made by hour hand with respect to 12:00 in h hours and m minutes.
Calculate the angle made by minute hand with respect to 12:00 in h hours and m minutes.
The difference between two angles is the angle between two hands.

Calculation:

Hour Hand:
- In 12 hours 360º, then 1hour = 30º
- In 720 minutes 360º, then 1min = 0.5º
- Angle travelled by Hour Hand = (h*30) + (m*0.5)
Minute Hand:
- In 60 min 360º, then 1min = 6º
- Angle travelled by Minute Hand = (m*6)

Implementation:

def clock_angle(hours, minutes):
    h = hours%12
    m = minutes%60

    hour_hand_angle = h*30 + m*0.5
    minute_hand_angle = m*6

    angle = abs(hour_hand_angle-minute_hand_angle)

    return min(angle, 360-angle)



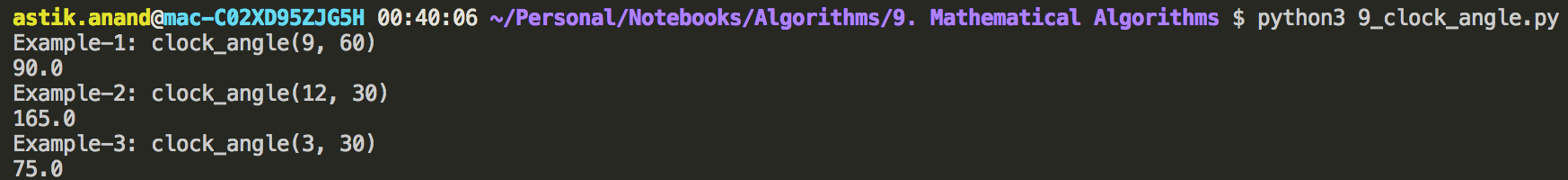
print("Example-1: clock_angle(9, 60)")
print(clock_angle(9, 60))

print("Example-2: clock_angle(12, 30)")
print(clock_angle(12, 30))

print("Example-3: clock_angle(3, 30)")
print(clock_angle(3, 30))

Output:

clock_angle_problem_output

Complexity:

Time: O(1)

9. Smallest number whose digit multiply to give number N

Problem:

Given a number ‘n’, find the smallest number ‘p’ such that if we multiply all digits of ‘p’, we get ‘n’.

The result ‘p’ should have minimum two digits.

Examples:

Input: n = 36 Output: p = 49 // Note that 4*9 = 36 and 49 is the smallest such number

Input: n = 100 Output: p = 455 // Note that 455 = 100 and 455 is the smallest such number

Input: n = 7 Output: p = 17 // Note that 1*7 = 7

Input: n = 13 Output: Not Possible

Approach:

Case 1: n < 10
- When n is smaller than n, the output is always n+10.
- Example: n = 7, output is 17. For n = 9, output is 19.
Case 2: n >= 10
- Find all factors of n which are between 2 and 9 (both inclusive).
- The idea is to start searching from 9 so that the number of digits in result are minimized.
- Example: 9 is preferred over 33 and 8 is preferred over 24.
- Store all found factors in an array, the array would contain digits in non-increasing order, so finally print the array in reverse order.

Implementation:

def smallest_num_digit_multiply_to_n(n):
    if(n<10):
        return 10+n
    
    # p is to store the digits
    p = ""
    for i in range(9, 1, -1):
        while(n%i==0):
            p += str(i)
            n = int(n/i)
    
    # If n is still grteater than 10
    if(n>10):
        return "Not Possible"
    
    # Reverse the p now.
    p = p[::-1]

    return p
            


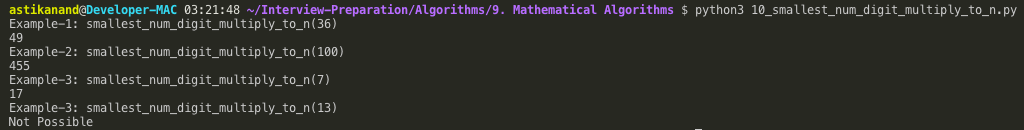
print("Example-1: smallest_num_digit_multiply_to_n(36)")
print(smallest_num_digit_multiply_to_n(36))

print("Example-2: smallest_num_digit_multiply_to_n(100)")
print(smallest_num_digit_multiply_to_n(100))

print("Example-3: smallest_num_digit_multiply_to_n(7)")
print(smallest_num_digit_multiply_to_n(7))

print("Example-3: smallest_num_digit_multiply_to_n(13)")
print(smallest_num_digit_multiply_to_n(13))

Output:

smallest_number_multiply_to_N_output

Complexity:

Time: O(1)

10. Birthday Paradox***

Q1. How many people must be there in a room to make the probability 100% that at-least two people in the room have same birthday?

Answer: 367 (since there are 366 possible birthdays, including February 29).

The above question was simple, let us see the below question.

Q2. How many people must be there in a room to make the probability 50% that at-least two people in the room have same birthday?

Answer: 23

The number is surprisingly very low. In fact, we need only 70 people to make the probability 99.9 %.

Generalized Formula

What is the probability that two persons among n have same birthday?

Implementation:

import math

def birthday_paradox_find_n(probability):
    print (math.ceil(math.sqrt(2*365*(math.log(1/(1-probability))))))


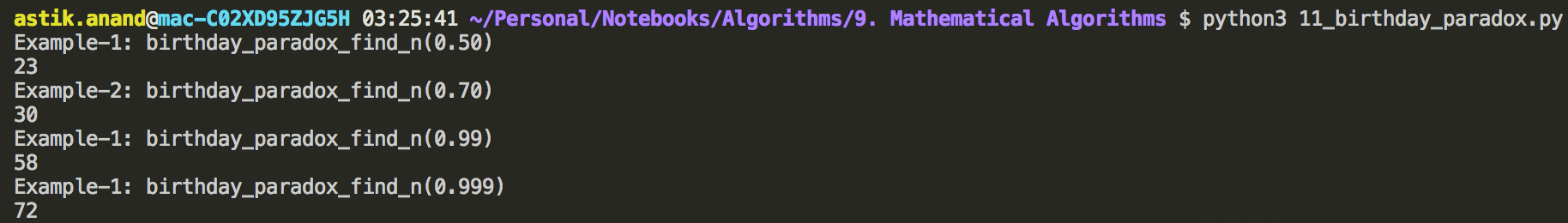
print ("Example-1: birthday_paradox_find_n(0.50)")
birthday_paradox_find_n(0.50)

print ("Example-2: birthday_paradox_find_n(0.70)")
birthday_paradox_find_n(0.70)

print ("Example-1: birthday_paradox_find_n(0.99)")
birthday_paradox_find_n(0.99)

print ("Example-1: birthday_paradox_find_n(0.999)")
birthday_paradox_find_n(0.999)

Output:

birthday_paradox_problem-output

Complexity:

Time: O(1)

← Previous: Bit Algorithms

Next: Geometric Algorithms →

Mathematical Algorithms

Standard Mathematical Algorithm Problems

1. Lucky Numbers***

What are lucky numbers?

Problem:

Algorithm:

Implementation:

Complexity:

2. Square Root - Babylonian Method***

Problem:

Algorithm:

Implementation:

Complexity:

3. Sieve of Eratosthenes

Problem:

Algorithm:

Implementation:

Complexity:

4. Next Smallest Palindrome***

Problem:

Implementation:

Complexity:

5. Prime Factors

Problem:

Algorithm:

Implementation

Complexity:

6. Trailing Zeroes in Factorial

Problem:

Approach:

Implementation:

Complexity:

7. Next Greater with Same Digits***

Problem:

Algorithm:

Implementation:

Complexity:

8. Clock Angle Problem***

Problem:

Approach:

Calculation:

Implementation:

Complexity:

9. Smallest number whose digit multiply to give number N

Problem:

Approach:

Implementation:

Complexity:

10. Birthday Paradox***

Q1. How many people must be there in a room to make the probability 100% that at-least two people in the room have same birthday?

Q2. How many people must be there in a room to make the probability 50% that at-least two people in the room have same birthday?

Generalized Formula

Implementation:

Complexity:

Subscribe !

Connect to me through Social Networks !

Contact Me !